
Bioinformatic tools were used to identify genetic signatures involving specific protein domains or long terminal repeat (LTR) transcription factor sites within co-linear viral protein R (Vpr), trans-activator of transcription (Tat), or LTR sequences that were preferentially associated with X4 or R5 Env-V3 sequences. The in silico position-specific scoring matrix (PSSM) algorithm was used to define distinct groups of X4 and R5 sequences based solely on sequences in Env-V3. Specific adaptive changes associated with X4 and R5 viruses were identified in co-linear viral sequences beyond the Env-V3. Additionally, R5 viruses have been associated with viral transmission and CNS disease and are also more prevalent during HIV-1 disease. By comparison, the CCR5-utilizing (R5) viruses have a greater preference for cells of the monocyte-macrophage lineage however, while R5 viruses also display a propensity to enter and replicate in T cells, they infect a smaller percentage of CD4 + T cells in comparison to X4 viruses. CXCR4-utilizing (X4) viruses preferentially, but not universally, infect CD4 + T cells, generating high levels of virus within activated HIV-1-infected T cells that can be detected in regional lymph nodes and peripheral blood.
#LINEAR SEQUENCES FREE#
If you need someone to explain this method to you in person, book in a free taster session here.įor even more linear nth term questions, try our nth term worksheet.The adaptation of human immunodeficiency virus type-1 (HIV-1) to an array of physiologic niches is advantaged by the plasticity of the viral genome, encoded proteins, and promoter. Have a go at finding the nth term of each of these sequences and put your answers in the comments or email them to and I’ll let you know if you got them right.
#LINEAR SEQUENCES HOW TO#
If you are studying the higher tier, you can read my guide on how to find the nth term of a quadratic sequence.īelow are 10 linear sequences. So there is an introduction to the nth term and how to find the nth term of a linear sequence. N = 1 -3×1 + 14 = 11 this matches our sequence Let’s check the first three terms just to be sure… If the number you get is not an integer, then this means the number is not in the sequence.

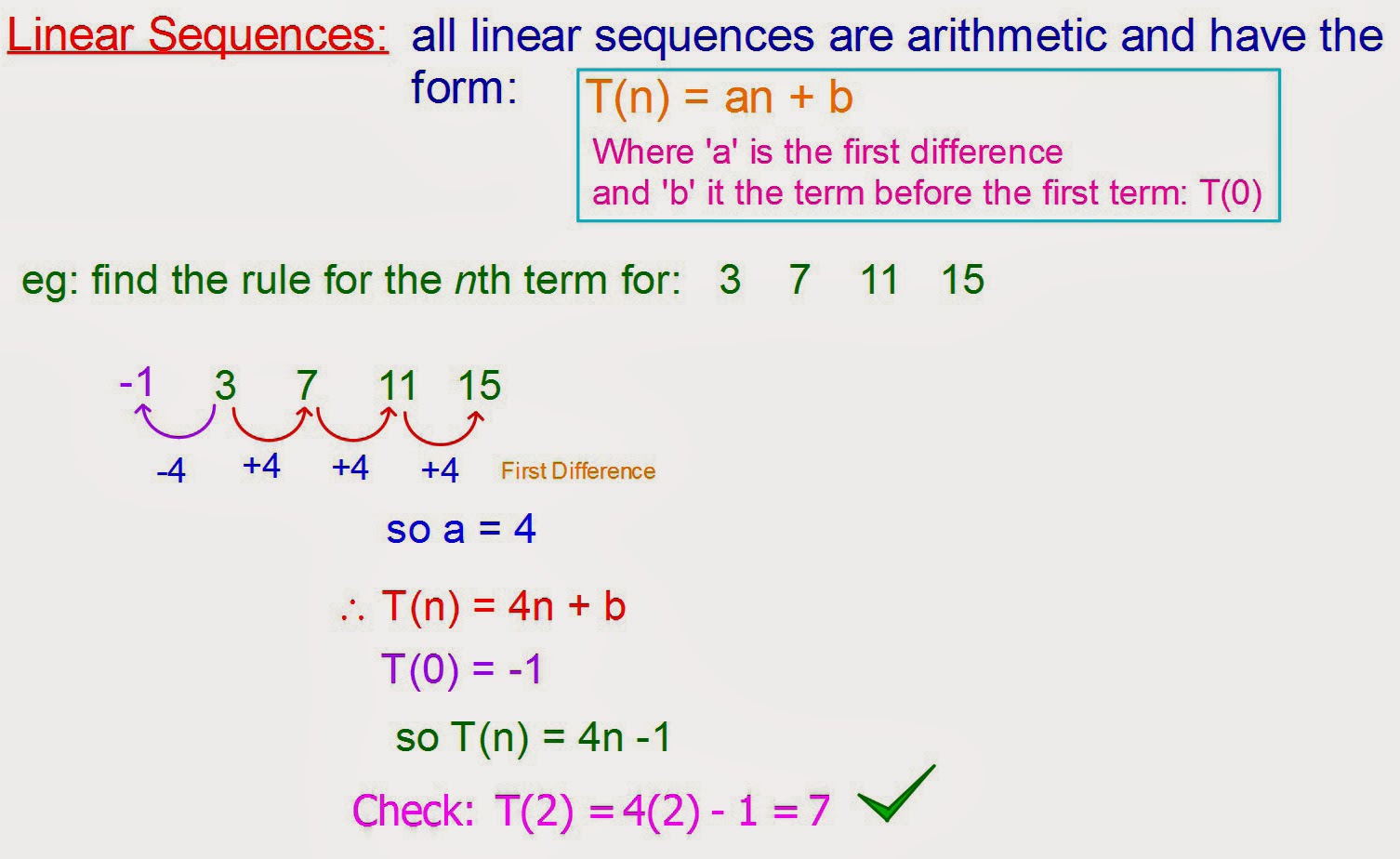
If the number you get is an integer, then this means the number is in the sequence. It makes sense to say that 665 is the 100 th term in a sequence, but not that it is the 100.6 th term in the sequence. However, if we don’t get a whole number, this makes no sense. And then solve it! This will basically do the opposite of what we did in the previous example – it will tell us what number in the sequence 665 is… if the number we get is a whole number, that will make sense. Let’s say I was asked “Is 665 in the sequence?”.Īgain, without the nth term, the only way to do this would be by writing out the sequence until we get to 665 and see whether it appears… but that’s going to take even longer than the last question!Īgain, the nth term can make this a lot easier and quicker for us.Īll we need to do is take our nth term formula, and make it equal to the number we are testing (in this case it’s 665). Let’s say we are still looking at the same sequence – 5, 9, 13, 17, … Much quicker than writing out 200 terms I’m sure you would agree! So in a matter of seconds we know that the 200 th term in the sequence is 801. With the nth term, you can get it instantly. If you had never heard of the nth term before, the only way you would know how to do this is to continue writing out the sequence until you get to the 200 th term… which would be time-consuming to say the least! So it is fairly easy to find the next few terms in the sequence.īut where the nth term becomes really useful is if I asked you to find the 200 th term in the sequence. You can clearly see here that the sequence we have generated is linear and goes up by 4 each time. So we know the sequence starts 5, 9, 13, 17. I can find the 2 nd, 3 rd and 4 th terms as well too.

Therefore I know the first term in the sequence is 5. If I wanted to find the 1 st term in the sequence, I can do that using the nth term. So let’s say a sequence has nth term 4n + 1.

The nth term is a formula in terms of n that will find any term in the sequence that you want. What is the nth term, and why is it useful?įirst of all, let me explain what the nth term of a sequence is. This is a relatively simple process, but is incredibly useful. Following on from the last blog on identifying different types of sequences, in this blog I will show you how to find the nth term of a linear sequence.


 0 kommentar(er)
0 kommentar(er)
